
In this problem, students understand: The top layer will have one basketball. Making a number table can help you see patterns more clearly.

Sometimes you can solve a problem just by recognizing a pattern, but more often you must extend the pattern to find the solution. This may require students to read the problem several times or put the problem into their own words. This involves identifying the key pieces of information needed to find the answer. Then students can circulate around the room to read each group's solution.ĭemonstrate that the first step to solving a problem is understanding it.

Or, students can explain their solutions in writing, and the teacher can display the solutions. After finding a solution, each group can present it to the class, explaining how they reached their solution and why they think it is correct. Using cooperative learning groups to find solutions to problems helps students verbalize their thinking, brainstorm ideas, discuss options, and justify their positions. For example: If you build a four-sided pyramid using basketballs and don't count the bottom as a side, how many balls will there be in a pyramid that has six layers? Introduce a problem to students that requires them to find the pattern in order to solve the problem.
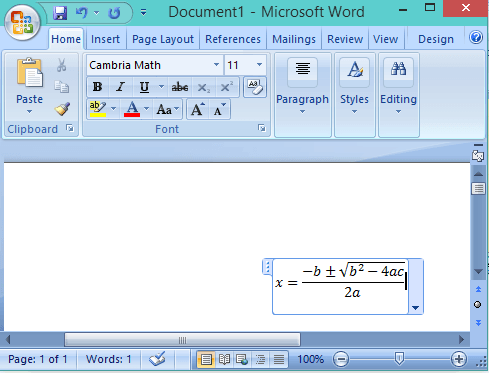
The Find a Pattern strategy can be used to solve many math problems and can be used in combination with many other strategies, including make a table, make a list, or simplify the problem. For example, yellow circle, red square, green triangle, yellow circle, red square, green triangle, and so on.ĭiscovering patterns can help students learn multiplication facts when they notice that 4 x 7 is the same as 7 x 4, and that all numbers in the 10s column end with a zero. Younger students often discover and continue using patterns that employ geometric shapes. Patterns are often introduced to students without the context of a word problem as in the following example: "Find a pattern in this sequence, explain how it works, and use that pattern to predict the next four numbers. How many lockers will be open at the end?įor the answer, go to: The Locker Problem from the Math Doctors Why Is It Important? This continues until every student has had a turn.
The first student opens all 1000 lockers next, the second student closes lockers 2, 4, 6, 8, 10, and so on up to locker 1000 the third student changes the state (opens lockers that are closed, closes lockers that are open) of lockers 3, 6, 9, 12, 15, and so on the fourth student changes the state of lockers 4, 8, 12, 16, and so on. The following problem can be solved by finding a pattern: There are 1000 lockers in a high school with 1000 students. Students look for items or numbers that are repeated, or a series of events that repeat. Problem Solving: Find a Pattern What Is It?įinding a Pattern is a strategy in which students look for patterns in the data in order to solve the problem.


 0 kommentar(er)
0 kommentar(er)
